Analysis of chaos in Willamowski-Rossler reaction.

In this project, which was done at the end of a Dynamical Systems course, I explored the chaotic behavior of the Willamowski-Rossler system, which models a series of autocatalytic chemical reactions. I didn’t delve into the chemistry; the important part for me was that the equations produced chaotic behavior. I analyzed the chaotic system and discussed some parameters that affect it. The full project can be found here.


The WR equations:

x˙=k1a1x−k−1x2−k2xy+k−2y2−k4xzy˙=k2xy−k−2y2−k3a5y+k−3a2z˙=k5a4z−k−5z2−k4xz+k−4a3\begin{align} \dot{x} &= k_1a_1x - k_{-1}x^2 -k_2xy +k_{-2}y^2 - k_4xz \\ \dot{y} &= k_2xy - k_{-2}y^2 -k_3a_5y+k_{-3}a_2 \\ \dot{z} &= k_5a_4z-k_{-5}z^2-k_4xz+k_{-4}a_3 \end{align}

These equations were found to have an attracting loop:

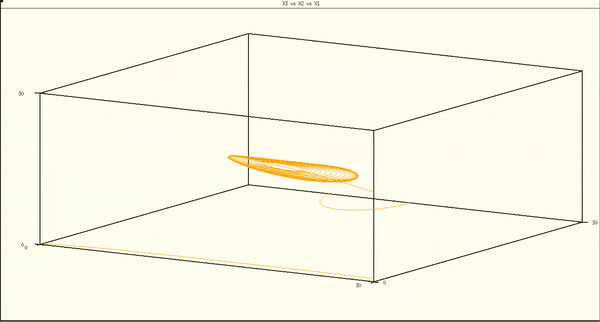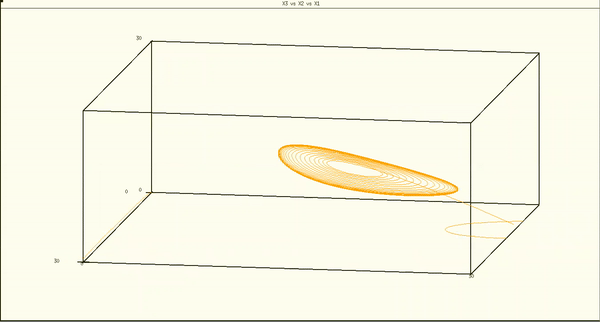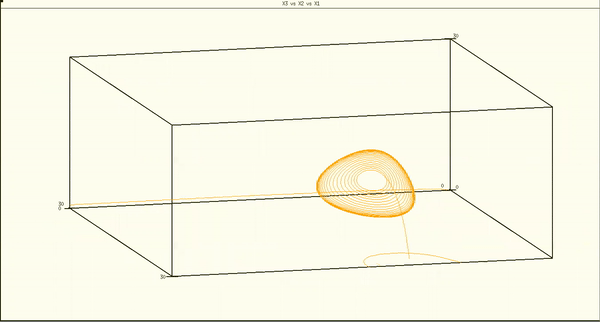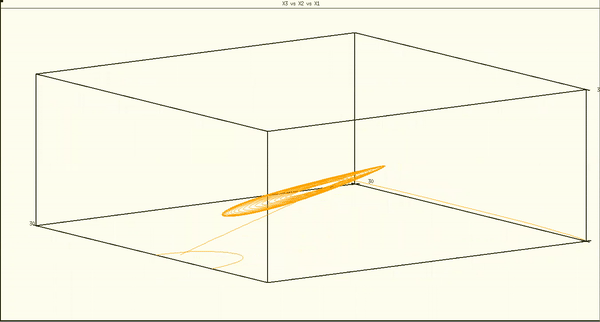
Also, under different parameters, they give rise to this beautiful strange attractor:

I used Python and the software XPPAUT to investigate the synchronization between two identical WR systems and the dependence of the final values on the initial conditions.

I found that the Willamowski-Rossler system exhibits fast synchronization, meaning that the two systems synchronize quickly and maintain synchronization even when the coupling strength is weak.


Next, I examined the dependence of the final values on specific parameters. I focused on k−2k_{-2}, which describes the rate of the reverse reaction and is the coefficient of the nonlinear term y2y^2 in the chaotic equations. Without it, we are diminishing the reverse reaction and therefore changing the whole system. I found that it has a bifurcation:

Overall, this project provided an amazing glimpse into the chaotic behavior of the Willamowski-Rossler system and chaos theory. It was also the first academic project that I did; I enjoyed researching and writing in LaTeX for the first time. As a result of this project, I worked with my advisor on two additional projects.